|
VMG—Velocity Made Good
by Lester Gilbert
VMG is the theory behind finding the pointing angle that
maximizes your progress towards the windward mark. It isn’t the angle at which
your boat sails the fastest, and it may not be the highest angle at which you
can point, if you want to. We’ll need it, along with the course theorem, to find
out the optimum pointing angle for maximum VMG.
To start, we’ll plot a graph of our boat speed at various
headings relative to the true wind. We’ll do this as a “radar” or “polar” chart
(hereinafter referred to as just polar)
and an example is shown in Figure 1. At a heading of 30 degrees, or 330 degrees,
it is the same, our boat makes about 1 m/s. At a heading of 90 degrees (and 270
degrees) our boat makes almost 1.5 m/s. At angles closer to the wind than 30
degrees, our boat speed decreases precipitously and goes to
zero. The polar of our boat’s
performance has characteristic lobes of highest speeds at reaching headings.
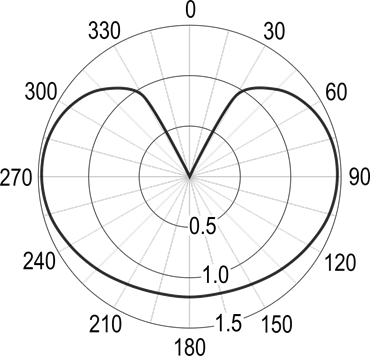
Figure 1. Polar chart of
boat speed for various headings
This polar becomes very useful in telling us the best
heading, which gets us to the windward mark in the least time; we simply find
that point on the polar that is closest to the mark. This is illustrated in
Figure 2, where the dotted line is the line we can draw that is closest to the
mark, while just touching the polar.

Figure 2. Optimum heading
for highest VMG
In our example, the point at which this closest line touches
the polar corresponds to a heading of 39 degrees. This is the optimum heading,
which gives us the best “velocity made good,” or VMG, towards the windward mark.
At this heading, reading off the polar, our boat speed is 1.13 m/s, and our VMG
is 0.88 m/s.
The polar also tells us what happens if we pinch and sail
closer to the wind than the heading that optimizes the VMG. For example, if we
point at a heading of 30 degrees, the polar tells us that boat speed drops to
0.95 m/s, and that although we are pointing better towards the mark, our VMG has
dropped to 0.82 m/s. Figure 3 illustrates this and also illustrates what happens
if we foot, that is, sail lower but with higher boat speed.

Figure 3. Sailing high and
sailing low
In our example, let’s suppose we foot to a heading of 50
degrees,
where the boat sails faster at 1.28 m/s. It turns out that our VMG has
dropped to 0.82 m/s, the same VMG as we’d get sailing at 30. Although the boat
is sailing much faster, it is just as slow getting to the mark.
It is interesting to look at the dotted VMG line of Figure 2
and to notice that in fact there is a region of the polar where VMG is within
0.05 percent of maximum, somewhere between headings of 35 and 42 degrees. If the
optimum heading under perfect conditions is 39 degrees, and pinching in order to
point at 30 degrees is significantly slower, we now know we are finely balanced
on a knife edge. Maybe we could pinch to 35 degrees, but after that we are
losing out. Those here, who can tell if they are sailing at 30 instead of 35
degrees—hands up? I thought not…
On the other hand, the knife edge is somewhat blunter when
footing and sailing low. Maybe we could foot to 42 degrees instead of trying to
maintain 39 degrees, particularly if we have waves or gusty wind. Footing at 50
degrees is as bad as pointing at 30 for VMG, but we are much less likely to lose
out, and may even gain. A wave or a heading gust at 30 can trip us over the
cliff and kill both boat speed and VMG, whereas the same event at 50 would
merely marginally increase boat speed and marginally lower VMG.
Our final challenge is to trim the sails to give us the
balance of lift and drag we need at the heading we wish to sail. Are we going to
maximize drive? Maximize efficiency? Or something else?
Acknowledgements
Graham Bantock made some helpful comments on an earlier draft
of the article.
|
.htm_cmp_lghome010_bnr.gif)